Forces between currents
When a current flows in a wire a magnetic field
is produced around the wire. If two conductors are placed close together then the field of one
wire affects the other and a force exists between the two wires.

This may be demonstrated simply by using the apparatus
shown in Figure 1, where a direct current of some 5 A is allowed to flow along two pieces of
cooking foil. When the currents in the two pieces of foil are in the same direction the strips of foil
attract each other and when they are in opposite directions they repel each
other.
The magnetic fields for the two wires are shown in Figure 2(a)
for currents in the same direction and 2(b) for currents in opposite directions. Points where there
is a zero resultant field are known as neutral points.

The force between the conductors is directly proportional to the current flowing in them and this therefore forms the basis for a definition of the
ampere.
The Ampere is defined as the constant current which, when flowing in two infinitely long, straight and parallel conductors of negligible cross section placed 1 m apart in a vacuum causes a force between them of 2x10-7 N per metre of their length.
The value of μo
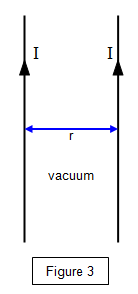
Consider the force of attraction between two infinitely long wires, each
carrying a current I (Figure 3).
The field B at one wire due to the other wire is:
B =
μ
oI/2πr
where r is
the distance between the two wires. The force F on a length L of this wire is therefore
Force (F) between two currents = BIL = μoI2L/2πr
If the wires are carrying a
current of 1 A and are 1 m apart in a vacuum and we consider unit length, then by the definition
of the ampere we have:
r = 1m I=1A F= 2x10
-7N
Therefore:
Permeability of free space (μo) = 4π x 10-7 Hm-1
Example problems
Example 1
Calculate the magnetic flux density at the centre of a solenoid of 2000 turns and 50 cm long if a current of 0.5 A flows in the coil.
B = moNI/L = [4πx10-7 x 2000 x 0.5]/0.5 = 2.5 x 10-3T
Example 2
What current must flow in an infinitely long straight wire to give a flux density the same as the above at 0.3 m from the wire?
B = μoI/2πr
Therefore I = 2.5x10-3x2πx0.3 = 3570 A!
A VERSION IN WORD IS AVAILABLE ON THE SCHOOLPHYSICS USB




 Consider the force of attraction between two infinitely long wires, each
carrying a current I (Figure 3).
Consider the force of attraction between two infinitely long wires, each
carrying a current I (Figure 3).